9th Maths 4.3

NCERT Class 9th solution of Exercise 4.1

NCERT Class 9th solution of Exercise 4.2

NCERT Class 9th solution of Exercise 4.4

NCERT Class 9th Maths Projects

Exercise 4.3

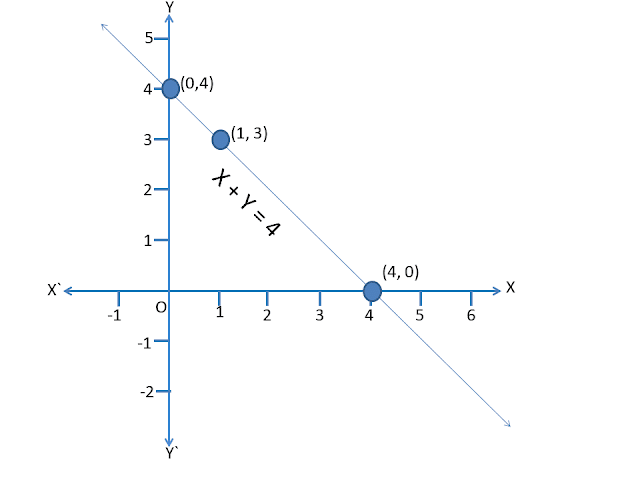
Q1. Draw the graph of each of the following linear equations in two variables :

i) `x+y=4` ii) `x-y=2` iii) `y=3x` iv) `3=2x+y`

Sol. :

i) Given equation `x+y=4` then `y=4-x`

When `x=0`

`y=4-0`

`y=4`

when `x=1`

`y=4-1`

`y=3`

when `y=0`

`0=4-x`

`x=4`

X	0	1	4
y	4	3	0

ii) Given equation `x-y=2` then `y=x-2`

when `x=0`

`y=0-2`

`y=-2`

when `x=1`

`y=1-2`

`y=-1`

when `y=0`

`0=x-2`

`x=2`

X	0	1	2
y	-2	-1	0

iii) Given equation `y=3x`

When `x=0`

`y=3(0)`

`y=0`

when `x=1`

`y=3(1)`

`y=3`

when `x=-1`

`y=3(-3)`

`y=-3`

X	0	1	-1
y	0	3	-3

iv) Given equation `3=2x+y` then `y=3-2x`

when `x=0`

`y=3-2(0)`

`y=3-0`

`y=3`

when `x=1`

`y=3-2(1)`

`y=3-2`

`y=1`

when `y=0`

`0=3-2x`

`2x=3`

`x=frac(3)(2)`

X	0	1	3/2
y	3	1	0

Q2. Give the equations of two lines passing through `(2,14)`. How many more such lines are there, and why?

Answer :

The equations of lines passing through `(2, 14)` are `x+y=16` and `7x-y=0`.

There are infinitely many lines that can be drawn through a point.

Q3. If the point `(3, 4)` lies on the graph of the equation `3y=ax+7`, find the value of `a`.

Sol. :

Given equation `3y=ax+7` and point `(3, 4)`

then the value of `a`

`3(4)=a(3)+7`

`12=3a+7`

`3a=12-7`

`3a=5`

`a=frac(5)(3)`

Answer :

The required value of `a=frac(5)(3)`.

Q4. The taxi fare in a city is as follows; For the first kilometre, the fare is Rs 8 and for the subsequent distance it is Rs. 5 per kilometre. Taking the distance covered as x km and total fare as Rs y, write a linear equation for this information, and draw its graph.

Sol. :

Let total distance `=x` km.

fare for Ist km     `=₹8`

the subsequent distance `=(x-1)` km

rate of fare of subsequent distance `=₹5` per km

the fare of subsequent distance `=₹5(x-1)`

Total fare `y=x+5(x-1)`

`y=8+5x-5`

`y=5x+3`

Answer :

The required linear equation is `y=5x+3`

and draw the graph of the linear equation

when `x=0`

`y=5(0)+3`

`y=3`

when `x=-1`

`y=5(-1)+3`

`y=-5+3`

`y=-2`

x	0	-1
y	3	-2

Q5. From the choice given below, choose the equation whose graphs are given in figure (a) and (b)

for figure (a),

i) `y=x`

ii) `x+y=0`

iii) `y=2x`

iv) `2+3y=7x` 

for figure (b), 

i) `y=x+2`

ii) `y=x-2`

iii) `y=-x+2`

iv) `x+2y=6`

Sol. : for figure (a)

There are two points `(-1, 1)`, and `(1, -1)`

i) `y=x`

`y-x=0`

`1-(-1)=0`

`1+1=0`

`2≠0

and 

`-1-1=0`

`-2≠0`

ii) `x+y=0`

`-1+1=0`

`0=0`

and

`1+(-1)=0`

`1-1=0`

`0=0`

iii)`y=2x`

`2x-y=0`

`2(-1)-1=0`

`-2-1=0`

`-3≠0`

and

`2(1)-(-1)=0`

`2+1=0`

`3≠0`

iv)`3+3y=7x`

`7x-3y=-3`

`7(-1)-3(1)=-3`

`-7-3=-3`

`-10≠-3`

and

`7(1)-3(-1)=-3`

`7+3=-3`

`10≠-3`

Answer :

The correct option (ii)

For figure (b)

There are two points `(0, 2)` and `(2, 0)`

i) `y=x+2`

`y-x=2`

`2-0=2`

`2=2`

and 

`0-2=2`

`-2≠2`

ii) `y=x-2`

`x-y=2`

`0-2=2`

`-2≠2`

and

`2-0=2`

`2=2`

iii) `y=-x+2`

`y+x=2`

`2+0=2`

`2=2`

and

`0+2=2`

`2=2`

iv) `x+2y=6`

`0+2(2)=6`

`0+4=6`

`4≠6`

and 

`2+2(0)=6`

`2+0=6`

`2≠6`

Answer :

The correct option (iii)

Q6. If the work done by a body on application of a constant force is directly proportional to the distance travelled by the body, express this in the form of an equation in two variables and draw the graph of the same by taking the constant force as 5 units. Also read from the graph the work done when the distance travelled by the body is 

i) 2 units  ii) 0 unit

Sol. :

Let the work done by the body be y units 

and the distance travelled by the body be x units.

ATQ.

`y=5x`

When `x=0` then `y=5(0)=0`

When `x=-1` then `y=5(-1)=-5`

When `x=1` then `y=5(1)=5`

X	0	-1	1
y	0	-5	5

i) `x=2` units

`y=5(2)` units

`y=10` units.

ii) `x=0` unit

`y=5(0)` unit

`y=0` unit.

Answer :

i) `10` units ii) `0` unit.

Q7. Yamini and Fatima, two students of Class IX of a school, together contributed Rs. 100 towards the Prime Minister's Relief Fund to help the earthquake victims. Write a linear equation which satisfies this data. ( You may take their contributions as Rs. x and Rs. y) Draw the graph of the same.

Sol. :

Let the contribution of Yamini is Rs. `x`

and the contribution of Fatima is Rs. `y`

ATQ

`x+y=100`

`y=100-x`

When `x=0` then `y=100-0=100`

When `x=100` then `y=100-100=0`

When `x=50` then `y=100-50=50`

X	0	100	50
y	100	0	50

Q8. In countries like USA and Canada, temperature is measured in Fahrenheit, whereas in countries like India, it is measured in Celsius. Here is a linear equation that converts Fahrenheit to celsius:

`F=(frac(9)(5))C+32`

i) Draw the graph of the linear equation above using Celsius for x-axis and Fahrenheit for y-axis.

ii) If the temperature is 30°C, what is the temperature in Fahrenheit?

iii) If the temperature is 95°F, what is the temperature in Celsius?

iv) If the temperature is 0°C, what is the temperature in Fahrenheit and if the temperature is 0°F, what is the temperature is 0°F, what is the temperature in Celsius?

v) Is there a temperature which is numerically the same in both Fahrenheit and Celsius? If yes, find it.  

Sol. :

i) `F=(frac(9)(5))C+32`

When `C=0°C` then `F=(frac(9)(5))(0)+32=32°F`

When `C=-40°C` then `F=(frac(9)(5))(-40)+32=-40°F`

When `F=0°` then `C=(frac(5)(9))(0-32)=-17.8°C`

°C	0°	-40°	-17.8°
°F	32°	-40°	0°

ii) If `C=30°C` then `F=(frac(9)(5))(30)+32=86°F` 

Answer :

`F=86°F`

iii) `C=(frac(5)(9))(95-32)=35°C`

Answer :

`C=35°C`

iv) `F=(frac(9)(5))(0)+32=32°F`

and `C=(frac(5)(9))(0-32)=-17.8°C`

Answer :

`F=32°F` and `C=-17.8°C`

v) Yes this temperature is possible.

Let `C=F=x`

`F=(frac(9)(5))C+32`

`x=(frac(9)(5))x+32`

`5x=9x+160`

`x=-40°`

Answer :

`C=F=-40°`