8th Maths 6.4
Chapter 6
Squares and Square Roots
NCERT Class 8th solution of Exercise 6.1
NCERT Class 8th solution of Exercise 6.2
NCERT Class 8th solution of Exercise 6.3
Exercise 6.4
Q1. Find the square root of each of the following numbers by Division Method.
i) `2304`
ii) `4489`
iii) `3481`
iv) `529`
v) `3249`
vi) `1369`
vii) `5776`
viii) `7921`
ix) `576`
x) `1024`
xi) `3136`
xii) `900`
`text{Sol.}`
i) `2304`
.png "manysolution12.blogspot.com")
`text{Answer:}`
`sqrt2304 = 48`
ii) `4489`
.png "manysolution12.blogspot,com")
`text{Answer:}`
`sqrt4489 = 67`
iii) `3481`
.png "manysolution12.blogspot.com")
`text{Answer:}`
`sqrt3481 = 59`
iv) `529`
.png "manysolution12.blogspot.com")
`text{Answer:}`
`sqrt529 = 23`
v) `3249`
.png "manysolution12.blogspot.com")
`text{Answer:}`
`sqrt3249 = 57`
vi) `1369`
.png "manysolution12.blogspot.com")
`text{Answer:}`
`sqrt1369 = 37`
vii) `5776`
.png "manysolution12.blogspot.com")
`text{Answer:}`
`sqrt5776 = 76`
viii) `7921`
.png "manysolution12.blogspot.com")
`text{Answer:}`
`sqrt7921 = 89`
ix) `576`
.png "manysolution12.blogspot.com")
`text{Answer:}`
`sqrt576 = 24`
x) `1024`
.png "manysolution12.blogspot.com")
`text{Answer:}`
`sqrt1024 = 32`
xi) `3136`
.png "manysolution12.blogspot.com")
`text{Answer:}`
`sqrt3136 = 56`
xii) `900`
.png "manysolution12.blogspot.com")
`text{Answer:}`
`sqrt900 = 30`
Q2. Find the number of digits in the square root of each of the following numbers (without any calculation).
i) `64`
ii) `144`
iii) `4489`
iv) `27225`
v) `390625`
`text{Sol.}`
`text{We know that, A perfect square is of }``n``text{-digits,}``text{ then its square root will have }n/2``text{ digit if }``n``text{ is even or }(n + 1)/2 text{ if }n text{ is odd}`
i) `64`
`n = 2 text{ [n is even]}`
`= n/2`
`= 2/2`
`= 1`
`text{Answer:}`
`text{Number of digit in }sqrt64 = 1`
ii) `144`
`n = 3 text{ [n is odd]}`
`= (n + 1 )/2`
`= (3 + 1)/2`
`= 4/2`
`= 2`
`text{Answer:}`
`text{Number of digit in }sqrt144 = 2`
iii) `4489`
`n = 4 text{ [n is even]}`
`= n/2`
`= 4/2`
`= 2`
`text{Answer:}`
`text{Number of digit in }sqrt4489 = 2`.
iv) `27225`
`n = 5 text{ [n is odd]}`
`= (n + 1)/2`
`= (5 + 1)/2`
`= 6/2`
`= 3`
`text{Answer:}`
`text{Number of digit in }sqrt27225 = 3`
v) `390625`
`n = 6 text{ [n is even]}`
`= n/2`
`= 6/2`
`= 3`
`text{Answer:}`
`text{Number of digit in }sqrt390625 = 3`.
Q3. Find the square root of the following decimal numbers.
i) `2.56`
ii) `7.29`
iii) `51.84`
iv) `42.25`
v) `31.36`
`text{Sol.}`
i) `2.56`
.png "manysolution12.blogspot.com")
`text{Answer:}`
`sqrt2.56 = 1.6`
ii) `7.29`
.png "manysolution12.blogspot.com")
`text{Answer:}`
`sqrt7.29 = 2.7`
iii) `51.84`
.png "manysolution12.blogspot.com")
`text{Answer:}`
`sqrt51.84 = 7.2`
iv) `42.25`
.png "manysolution12.blogspot.com")
`text{Answer:}`
`sqrt42.25 = 6.5`
v) `31.36`
.png "manysolution12.blogspot.com")
`text{Answer:}`
`sqrt31.36 = 5.6`
Q4. Find the least number which must be subtracted from each of the following numbers so as to get a perfect square. Also find the square root of the perfect square so obtained.
i) `402`
ii) `1989`
iii) `3250`
iv) `825`
v) `4000`
`text{Sol.}`
i)
`text{By long division method}`
.png "manysolution12.blogspot.com")
`text{We get remainder 2.}`
`text{Its shows that }20^2 text{ is less than 402 by 2.}`
`text{2 is the least number which must be }``text{subtracted from the 402 to get a perfect square.}`
`text{Therefore perfect square is 402 - 2 = 400.}`
`text{And} sqrt400 = 20.`
`text{Answer:}`
`2; 20.`
ii)
`text{By long division method}`
.png "manysolution12.blogspot.com")
`text{We get remainder 53.}`
`text{Its shows that }44^2 text{ is less than 1989 by 53.}`
`text{53 is the least number which must be }``text{subtracted from the 1989 to get a perfect square.}`
`text{Therefore perfect square is 1989 - 53 = 1936.}`
`text{And} sqrt1936 = 44.`
`text{Answer:}`
`53; 44.`
iii)
`text{By long division method}`
.png "manysolution12.blogspot.com")
`text{We get remainder 1.}`
`text{Its shows that }57^2 text{ is less than 3250 by 1.}`
`text{2 is the least number which must be }``text{subtracted from the 3250 to get a perfect square.}`
`text{Therefore perfect square is 3250 - 1 = 3249.}`
`text{And} sqrt3250 = 57.`
`text{Answer:}`
`1; 57.`
iv)
`text{By long division method}`
.png "manysolution12.blogspot.com")
`text{We get remainder 41.}`
`text{Its shows that }28^2 text{ is less than 825 by 41.}`
`text{41 is the least number which must be }``text{subtracted from the 825 to get a perfect square.}`
`text{Therefore perfect square is 825 - 41 = 784.}`
`text{And} sqrt784 = 28.`
`text{Answer:}`
`41; 28.`
v)
`text{By long division method}`
.png "manysolution12.blogspot.com")
`text{We get remainder 31.}`
`text{Its shows that }63^2 text{ is less than 4000 by 31.}`
`text{31 is the least number which must be }``text{subtracted from the 4000 to get a perfect square.}`
`text{Therefore perfect square is 4000 - 31 = 3969.}`
`text{And} sqrt3969 = 63.`
`text{Answer:}`
`31; 63.`
Q5. Find the least number which must be added to each of the following numbers so as to get a perfect square. Also find the square root of the perfect square so obtained.
i) `525`
ii) `1750`
iii) `252`
iv) `1825`
v) `6412`
`text{Sol.}`
i)
`text{By division method}`
.png "manysolution12.blogspot.com")
`text{We get remainder 41.}`
`text{It shows that } 22^2 text{is less than 525 by 41.}`
`text{Next perfect square number is }23^2 = 529.`
`text{Hence, the number to be added is }``529 - 525 = 4.`
`text{Answer:}`
`4; 23.`
ii)
`text{By division method}`
.png "manysolution12.blogspot.com")
`text{We get remainder 69.}`
`text{It shows that } 41^2 text{is less than 1750 by 69.}`
`text{Next perfect square number is }42^2 = 1764.`
`text{Hence, the number to be added is }``1764 - 1750 = 14.`
`text{Answer:}`
`14; 42.`
iii)
`text{By division method}`
.png "manysolution12.blogspot.com")
`text{We get remainder 27.}`
`text{It shows that } 15^2 text{is less than 252 by 27.}`
`text{Next perfect square number is }16^2 = 256.`
`text{Hence, the number to be added is }``256 - 252 = 4.`
`text{Answer:}`
`4; 16.`
iv)
`text{By division method}`
.png "manysolution12.blogspot.com")
`text{We get remainder 61.}`
`text{It shows that } 42^2 text{is less than 1825 by 61.}`
`text{Next perfect square number is }43^2 = 1849.`
`text{Hence, the number to be added is }``1849 - 1825 = 24.`
`text{Answer:}`
`24; 43.`
v)
`text{By division method}`
.png "manysolution12.blogspot.com")
`text{We get remainder 12.}`
`text{It shows that } 80^2 text{is less than 6412 by 12.}`
`text{Next perfect square number is }81^2 = 6561 .`
`text{Hence, the number to be added is }``6561 - 6412 = 149.`
`text{Answer:}`
`149; 81.`
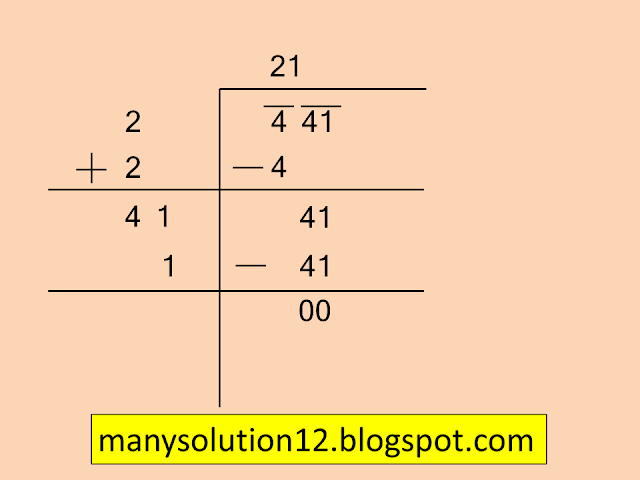
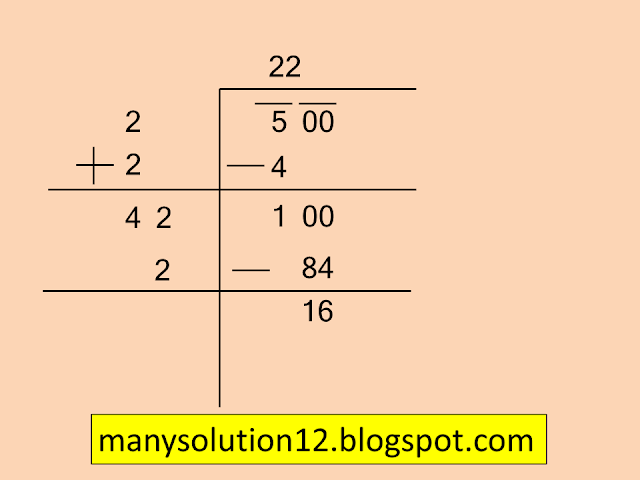
Q6. Find the length of the side of a square whose area is `441 text{m}^2`.
`text{Sol.}`
`text{Let the length of the side of a square is x}`
`text{Then}`
`text{Area of square = (Side)}^2`
`441 = text{(x)}^2`
`441 = text{x}times text{x}`
`441 = text{x}^2`
`text{x} = sqrt441`
`text{By long division method}`
`text{x} = 21`
`text{Answer:}`
`text{The length of the side of a square is 21 m}^2`.
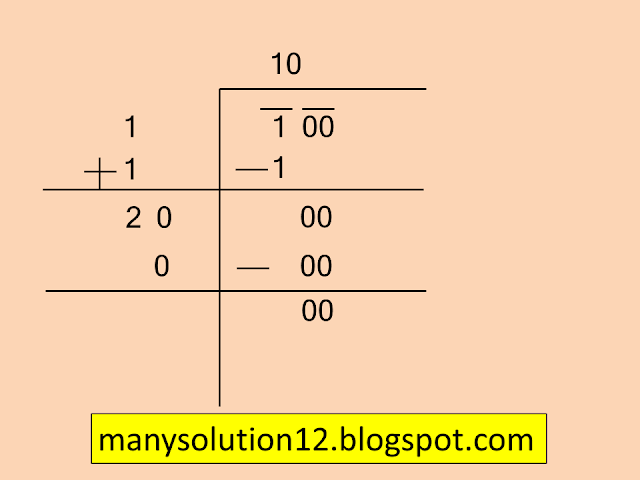
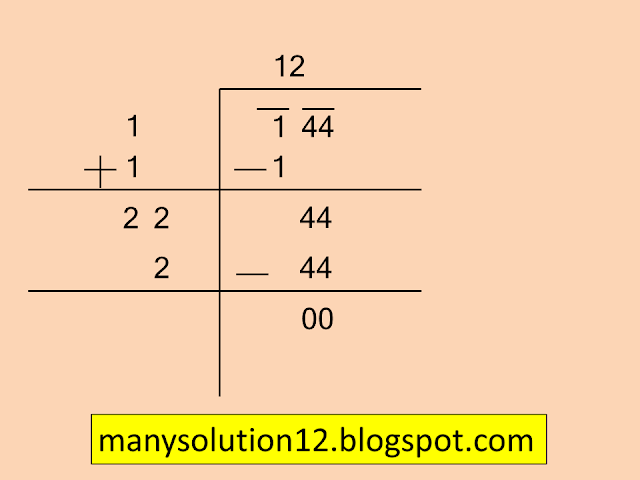
Q7. In a right triangle `text{ABC, } angle text{B} = 90^circ`.
a) If `text{AB = 6 cm, BC = 8 cm,}` find `text{AC}`
b) If `text{AC = 13 cm, BC = 5 cm,}` find `text{AB}`
`text{Sol.}`
a)
`text{In right }triangletext{ ABC}`
`text{By Pythagors Theorem}`
`text{AC}^2 = text{AB}^2 + text{BC}^2`
`text{AC}^2 = (6)^2 + (8)^2`
`text{AC}^2 = 36 + 64`
`text{AC}^2 = 100`
`text{AC} = sqrt100`
`text{By long division method}`
`text{AC} = 10`
`text{Answer:}`
`text{AC} = 10`.
b)
`text{In right }triangletext{ ABC}`
`text{By Pythagors Theorem}`
`text{AC}^2 = text{AB}^2 + text{BC}^2`
`text{AB}^2 = text{AC}^2 - text{BC}^2`
`text{AB}^2 = (13)^2 - (5)^2`
`text{AB}^2 = 169 - 25`
`text{AB}^2 = 144`
`text{AB} = sqrt144`
`text{By long division method}`
`text{AB} = 12`
`text{Answer:}`
`text{AB} = 12`.
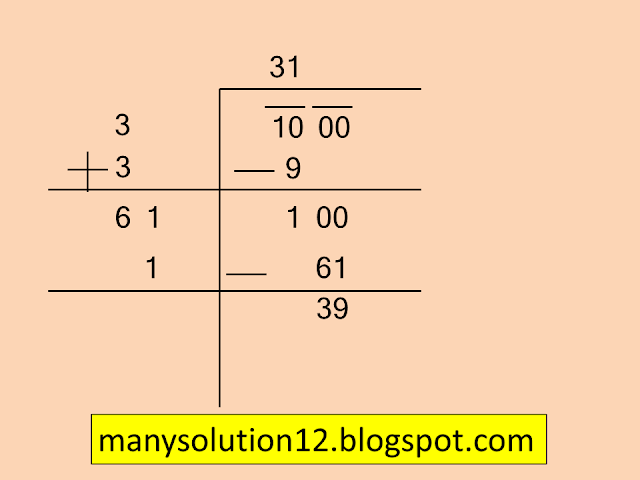
Q8. A gardener has `1000` plants. He wants to plant these in such a way that the number of rows and the number of columns remain same. Find the minimum number of plants he needs more for this.
`text{Sol.}`
`text{Let the number of rows be x}`
`text{and the number of columns be x}`
`text{Then x}times text{x} =1000`
`text{x}^2 = 1000`
`text{x} = sqrt1000`
`text{We find }sqrt1000 text{by long division method}`
`text{The remainder is 39}`
`text{This shows that the square of 31 is less than}`` 1000`
`text{Next perfect square number is 32 is equal}``text{ to 1024}`
`text{Hence, the number to be added is}``text{ 1024 - 1000 = 24}`
`text{Answer:}`
`text{The minimum number of plants he needs 24}`
Q9. There are `500` children in a school. For a P.T. drill they have to stand in such a manner that the number of rows is equal to number of columns. How many children would be left out in this arrangment.
`text{Sol.}`
`text{Let the number of rows be x}`
`text{and the number of columns be x}`
`text{Then x}times text{x } = 500`
`text{x}^2 = 500`
`text{x} = sqrt500`
`text{By long division method}`
`text{remainder is 16}`
`500 - 16 = 484`
`text{Answer:}`
`text{16 children would be left out in this}``text{ arrangment}`.
Comments